
Atomic, Molecular, and Optical Physics
1. Many-Body Excitations in Trapped Bose-Einstein Condensates
Excitation spectra of quantum systems are one of the widely used notions in our modern quantum world. They often allow one to explore the microscopic structure of different quantum objects, such as nuclei, atoms, molecules, and solids, and more recently, of Bose-Einstein condensates (BECs). Experimentally, owing to their controllability, excitations of BECs have widely been explored. Theoretically, excitation spectra of dilute BECs have amply been studied within Bogoliubov—de Gennes theory, which assumes the presence of a simple condensate and only a small amount of excitations and quantum depletion atop.
There are available experiments with BECs which have measured and identified excitations that cannot be described by the standard Bogoliubov—de Gennes theory. Our group develops and utilizes novel theoretical and computational methods, noticeably the multiconfigurational time-dependent Hartree for bosons (MCTDHB) method and its linear-response (LR-MCTDHB) atop, to describe and investigate such excitations.
2. Many-body Tunneling Processes of Bose-Einstein Condensates
Tunneling is a process which lies at the very heart of quantum mechanics. It is of general and wide interest and of importance in physics, chemistry, biology, and technology. Already the text-book example of single-particle tunneling under a potential barrier in one spatial dimension is wealth. Interactions between tunneling particles make it profoundly richer. Higher spatial dimensions open essentially unlimited possibilities.
Bose-Einstein condensates offer the opportunity to theoretically study tunneling processes in a synthetic yet highly controllable many-particle system. Our group investigates how interactions, trapping, topology, and dimensionality conspire to allow for novel many-particle tunneling phenomena and mechanisms in BECs, with core emphasis on the time-dependent description of the tunneling process.
3. Variance as a Sensitive Probe of Correlations in Interacting Bose Systems
Bose-Einstein condensates made of ultra-cold trapped bosonic atoms have become a central venue in which interacting many-body quantum systems are studied. The ground state of a trapped BEC has been proven to be 100% condensed in the limit of an infinite number of particles and at constant interaction parameter, i.e., when the product of the number of particles times the scattering length is fixed. The meaning of this result is that properties of the condensate, noticeably its energy per particle and density per particle, converge to those obtained by minimizing the Gross-Pitaevskii, mean-field energy functional. This naturally raises the question whether correlations are of any importance in this limit.
Our group explores, numerically and analytically, the variance of many-particle operators which can show, even in the infinite-particle limit and at constant interaction parameter, substantial deviation from the Gross-Pitaevskii, mean-field result, thus elucidating the presence of many-body correlations in this limit.
4. Solvable Many-Body Models of Bose-Einstein Condensates and Mixtures Thereof
Solvable many-body models offer the unique possibility to investigate quantum many-particles systems analytically. They can provide definite answers on questions arising from preliminary intuition, and complement investigations necessitating numerical tools. In the latter context, solvable many-body models are also instrumental in benchmarking numerical methods.
Our group develops and applies solvable models, chiefly by generalizing the harmonic-interaction model for mixtures of identical particles, to investigate fundamental properties of trapped mixtures at the inter-junction of many-body and Gross-Pitaevskii, mean-field physics, such as the energy, density and reduced density matrices, separability, variance, and uncertainty product.
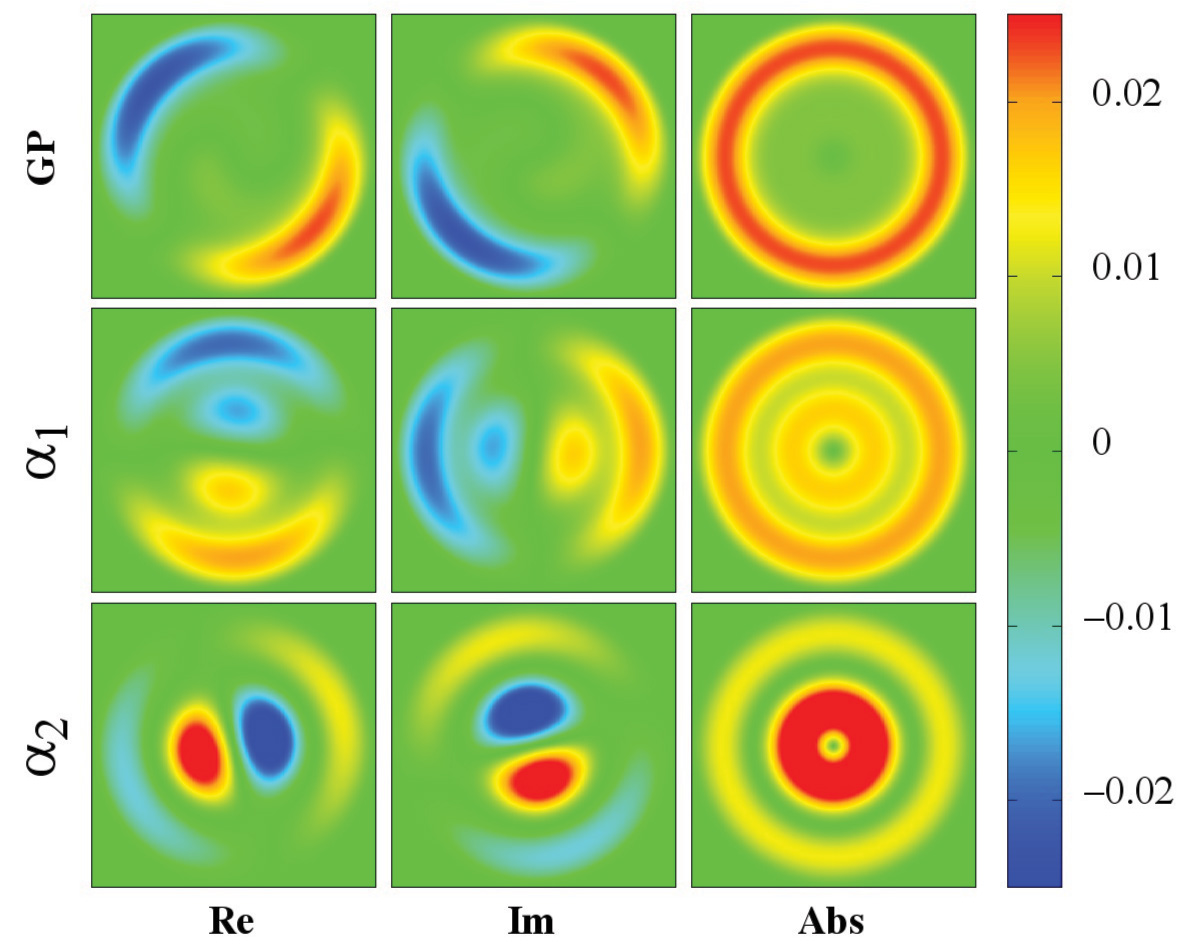
Figure 1: Tunneling and fragmentation of a BEC vortex in a circular trap. For more details see Phys. Rev. A 92, 043627 (2015). [LINK TO: https://doi.org/10.1103/PhysRevA.92.043627]
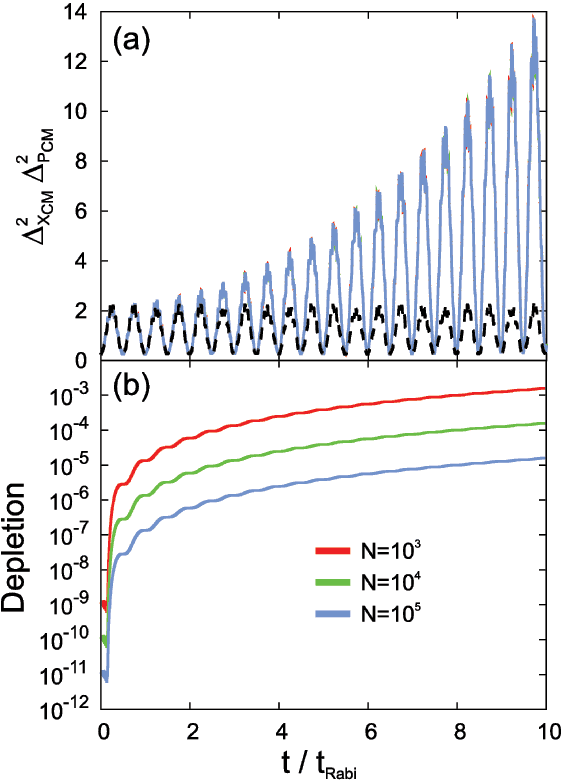
Figure 2: Center-of-mass uncertainty product of a BEC in a bosonic Josephson junction. For more details see Phys. Rev. A 93, 023605 (2016). [LINK TO: https://doi.org/10.1103/PhysRevA.93.023605]
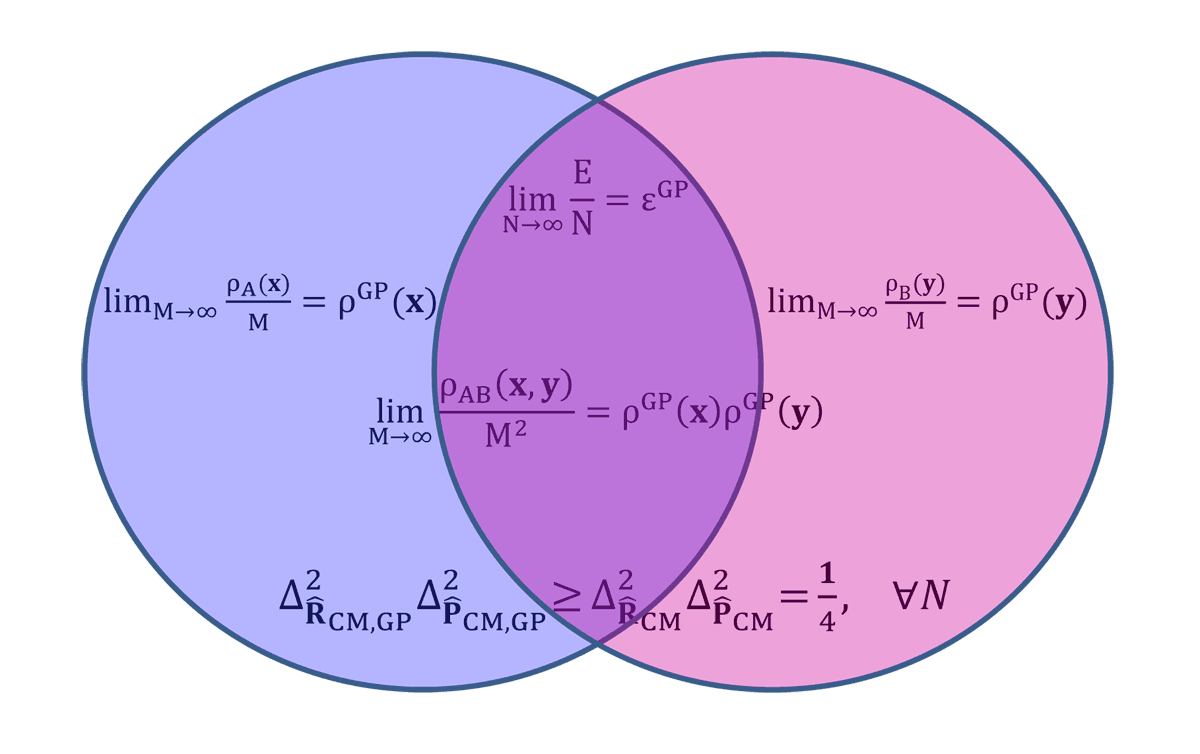
Figure 3: An artist’s view of variance in a trapped BEC mixture at the infinite-particle limit. For more details see Chem. Phys. 482, 362 (2017). [LINK TO: http://dx.doi.org/10.1016/j.chemphys.2016.07.011]
5. Quantum Diffusion in the coarse grained limit
Diffusion is ubiquitous in nature, both in classical and quantum systems. It is therefore pleasing that a complete nonequilibrium theory of diffusion exists — the macroscopic fluctuation theory. The macroscopic fluctuation theory captures the probability of fluctuations in nonequilibrium classical setup, in terms of two macroscopic quantities the diffusion and conductivity.
However, diffusion is not limited to classical physics. A generalization of the macroscopic fluctuation theory to the quantum regime promises to bring a paradigm shift in the study of nonequilibrium quantum dynamics; from detailed study of specific solvable models to a structured coarse grained approach almost at the level of equilibrium physics governed by Boltzmann weights. In recent years, it has been demonstrated that quantum diffusive systems, not corresponding to the macroscopic fluctuation theory description can be observed. Moreover, these systems exhibit universal structure that resembles the characteristics of the macroscopic fluctuation theory. It is one of the coveted goals to establish a coarse grained description of quantum diffusion at the level of generality of the macroscopic fluctuation theory.
6. Entanglement evaluation between a single agent to many body system
Ever since its discovery, entanglement has been one of the defining properties of quantum over classical systems. However, the last 20 years or so have witnessed a surge of interest in employing entanglement as a useful resource for quantum computing, quantum simulators and quantum metrology. This surge has led to numerous studies on the nature of the ground state entanglement in lattice systems and has led to the famous area law of the bipartite entanglement entropy.
Despite this large body of work, the properties of entanglement between a single agent to a many body system have not been as thoroughly explored. Furthermore it is unclear how large can the many body system be and still be entangled in a measurable way to the single agent. Recently, we have demonstrates that when a two site many body system is biased to have an overwhelming majority of particles in one site, both the Von Neumann entanglement entropy and the Logarithmic negativity show a typical power law in large R — the occupancy ratio between the highly occupied site to the depleted site. This is achieved in several limits for the ground state of a Hamiltonian system: the Bose-Hubbard model and for the steady state of a Lindblad system: the quantum asymmetric inclusion process.
Our results suggest that a large number of particles can be entangled to a single agent (as already demonstrated experimentally). Moreover, in some cases, the exponent of the power law is recovered analytically. The exponent is the critical parameter determining how large can the many body system be before entanglement becomes non-measurable. Furthermore, the power law decay suggest that ground states in our setup are unique. Their Von Neumann entanglement values are significantly lower than that of a typical pure state that grows logarithmically with the number of particles. This may suggest that the ground states are easier to access numerically, as is true for ground states following the area law in extended systems.



